Introduction to Integers in Mathematics
In Mathematics, we learn about various concepts. But all the concepts in Maths are related to numbers and their different forms that we are introduced with. The most common type of numbers which we learn are integers. These integers are positive numbers, negative numbers and even zero but they are not fractions.
Since, these integers are just the numerical values, therefore, it is easy to perform mathematical operations on them, such as addition and subtraction, multiplication and division. Hence, the integers also define the basicity of arithmetic operations.
What is Integer?
An integer is a type of real number that is not a fraction. These integers are positive and non-positive natural numbers including zero. For example, 8, 0, -8, are integers. But, ½, ¼, ⅔, ⅚, etc. are not integers.
Integers are usually denoted by capital letter ‘Z’.
Positive integers
The integers that are non-negative in nature or greater than zero are called positive integers.
The examples are 1,2,3,4,5,6,7,8,9,…etc.
Negative integers
The integers that are negative in nature or less than zero are called negative integers.
The examples are -1,-2,-3,-4,-5,-6,-7,-8,…etc.
Zero
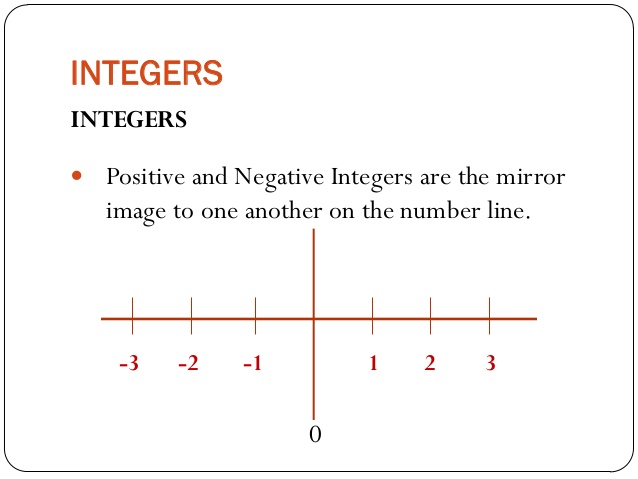
Zero is an integer that is neither negative nor positive. But 0 is a whole number. These integers are represented on the number line such that 0 is the center of the number, negative integers lie on the left hand side of 0 and positive integers lie on the right hand side of zero.
Z = {… -10, -9, -8, -7, -6, -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, 6, 7,8,9,10, …}
Thus, the integers extend on both sides of the number line till infinity.
Other Types of Integers
We can categorise the integers based on various concepts, such as;
- Consecutive integers
- Odd Integers
- Even Integers
In general all these types of integers are collectively called integers only. It is just the way of presentation that makes them different from each other. For example, the consecutive integers is the set of integers that differ by 1. If Z is an integer then its consecutive integers will be:
Z, Z+1, Z+2, Z+3,Z+4, and so on.
Example: If Z = 3, then the next three consecutive integers will be;
Z+1 = 3 + 1 = 4
Z + 2 = 3 + 2 = 5
Z + 3 = 3 + 3 = 6
The list of odd integers are 1,3,5,7,9,11,13,15, and so on. We can also say, these odd integers are odd consecutive integers, as they differ by 2. Similarly, the list of even integers are 2,4,6,8,10,12,14, and so on. Hence, these integers are also called even consecutive integers. We can see that odd integers and even integers resemble the general definition of odd and even numbers. Thus, integers are also simple numbers.
Properties of integers
The integers have the following properties:
- Integers are closed under addition, subtraction and multiplication.
- The change in order of two integers, while adding or multiplying, does not change the result.
- The regrouping of any three integers, while adding or multiplying, does not change the result.
- On adding 0 or multiplying by 1, the integer’s value remains unchanged
Conclusion
Integers are simply the numbers that are used to perform mathematical operations. We can add and subtract any two or more integers. Also, we can find the product of any two or more integers. The division method on integers is similar to division of natural numbers.
